MONTY HALL PROBLEM
Unit 2: FACTORIALS AND PERMUTATIONS. MONTY HALL PROBLEM.
Warm-up: Speaking.
1) What do you know about the Monty Hall problem?
2) Do you know any other mathematical assumptions or issues that you may want to share in class?
Follow-up 1: Reading comprehension.
Below is a reading comprehension. The text integrates the Monty Hall problem with key statistical vocabulary. Following the text, you'll find a series of activities to reinforce both the content and language skills.
READING:
The Curious Case of the Monty Hall Problem
Imagine you are a contestant on a popular game show. In this show, there are three closed doors. Behind one door is a shiny new car, and behind the other two are goats. The rules are simple: you choose one door, and then the host, who knows what is behind each door, opens one of the remaining doors to reveal a goat. Finally, you are given the opportunity to stick with your original choice or switch to the other unopened door.
At first glance, it might seem that there is a fifty-fifty chance of winning the car since only two doors remain. However, this is where the Monty Hall problem—a well-known probability puzzle—challenges our intuition. In fact, if you switch doors, your chance of winning the car increases to two-thirds.
This result is counterintuitive, and it is rooted in the concept of conditional probability. Initially, when you choose a door, there is a one-third probability that the car is behind that door and a two-thirds probability that the car is behind one of the other two doors. When the host reveals a goat behind one of these doors, the two-thirds chance does not vanish; rather, it is transferred entirely to the remaining door. Thus, by switching, you are more likely to win.
Let’s break down some of the key vocabulary used in this problem:
- Probability: The likelihood of an event occurring. In our example, the chance of picking the door with the car is a probability.
- Contestant: A person who participates in the game show.
- Host: The person who runs the show and reveals information; in this case, the host opens a door that hides a goat.
- Outcome: The result of an action or event. For example, the outcome can be either winning the car or ending up with a goat.
- Conditional probability: The probability of an event occurring given that another event has already occurred. Here, the chance of winning the car when you switch is a conditional probability based on the host’s action.
- Intuitive: What feels natural or seems obvious, even if it is not mathematically correct.
The Monty Hall problem serves as an excellent example to illustrate how our intuitive judgments can sometimes be misleading when it comes to probability. It is a fascinating topic not only because of its surprising outcome but also because it encourages us to examine the underlying assumptions of statistical reasoning.
In statistical studies and everyday situations, it is important to remember that events can be dependent on previous outcomes. For instance, the fact that the host always opens a door with a goat is not a random occurrence; it is a rule that alters the sample space (the set of all possible outcomes). Such rules help us understand that even in games of chance, our choices can significantly affect the final outcome.
To cut a long story short, Monty Hall problem can be used to explore other statistical ideas, such as the law of large numbers and the concept of expected value. These ideas are crucial for making informed decisions in both academic and real-world situations. Moreover, discussing problems like these can help improve one’s ability to communicate complex ideas in clear and precise English—a key skill in the field of statistics.
Activities:
Activity 1: True/False Questions
- The Monty Hall problem involves choosing one door out of three.
- Initially, the probability of the car being behind the chosen door is two-thirds.
- If the contestant switches their choice after the host opens a door, the probability of winning the car is two-thirds.
- The host’s action of revealing a goat is random.
- Conditional probability plays a significant role in solving the Monty Hall problem.
- The intuitive answer for this problem is that switching does not change the probability of winning.
Activity 2: Vocabulary Matching
Match the following words with their definitions:
VOCABULARY | DEFINITIONS |
a. Probability
| 1. The set of all possible outcomes of an event. 2. The likelihood or chance that a particular event will occur. 3. A person who takes part in a competition or game. 4. Dependent on a condition or previous event. 5. The result or consequence of an event or action.
|
Activity 3: Fill in the Blanks
Complete the sentences using words from the vocabulary list above (probability, contestant, outcome, conditional, sample space):
- In a game show, each __________ must decide whether to stick with their initial door choice or to switch.
- The __________ of winning the car increases when the player uses a switching strategy.
- The __________ after the host reveals a goat is that the remaining door has a higher chance of hiding the car.
- The chance of an event happening depends on its __________ context.
- The __________ for this problem includes the car being behind any of the three doors.
Activity 4: Discussion Questions
- Why do you think many people find the result of the Monty Hall problem counterintuitive?
- How does the concept of conditional probability help us understand real-world situations better?
- Can you think of a situation outside of a game show where understanding the sample space might change the outcome of a decision?
Follow-up: Listening.
1) Watch this video in class, pay attention to the explanations and then, try to answer the following questions:
1. The Monty Hall problem demonstrates a difficulty in understanding probabilities when:
(a) The prize is undesirable.
(b) The initial choice is difficult to make.
(c) The base assumptions of the situation change.
(d) The game show host is deceptive.
2. In the Monty Hall problem, after the host reveals a wrong option, switching your choice:
(a) Decreases your chances of winning to 33.3%.
(b) Keeps your chances of winning at 50%.
(c) Increases your chances of winning to 66.6%.
(d) Guarantees you will win the prize.
3. Why does the 100-curtain example make the Monty Hall problem more intuitive?
(a) It simplifies the probability calculation.
(b) It highlights the low probability of the initial guess being correct.
(c) It removes the element of chance entirely.
(d) It makes the host's actions seem less arbitrary.
4. The video criticizes the example of Nash Equilibrium in "A Beautiful Mind" because it is:
(a) Mathematically inaccurate.
(b) Too complex for a general audience.
(c) Needlessly sexist and insulting.
(d) Irrelevant to the film's plot.
5. At its simplest form, the Nash equilibrium theory proposes that there exists scenarios where:
(a) All participants benefit equally, regardless of strategy.
(b) All participants have an incentive to change their strategies.
(c) Competing participants who know the optimal strategies of all other participants have no incentive to unilaterally change their own standing in order to win an advantage over the others
(d) One participant always wins at the expense of others.
6. In the car analogy illustrating Nash equilibrium, the universal binary choice is:
(a) Both cars continue straight.
(b) Both cars swerve in the same direction.
(c) Both cars swerve in opposite directions.
(d) One car stops while the other proceeds.
7. The example of driver education and roadway conventions highlights the importance of:
(a) Individual freedom on the road.
(b) Public awareness of ideal strategies for shared success.
(c) The superiority of larger vehicles.
(d) A competitive driving environment.
8. The video suggests that Rogue States are a greater concern regarding nuclear weapons because:
(a) They are more likely to use them preemptively.
(b) They are less predictable and less likely to adhere to established strategies.
(c) They have more advanced nuclear technology.
(d) They are more likely to share nuclear technology with other countries.
9. Induced demand theory, as mentioned in the video, suggests that adding lanes to a highway:
(a) Always reduces traffic congestion.
(b) Has no impact on traffic patterns.
(c) Actually creates worse traffic problems.
(d) Only helps during peak hours.
10. The Soviet Union abandoned further testing of tritium bombs because:
(a) They were too expensive to develop.
(b) They violated international treaties.
(c) They realized there was no theoretical limit to the payload size of a tritium bomb and this would upset the Nash equilibrium of the nuclear powers.
(d) They were technologically inferior to American bombs.
11. Eugene Wigner's "unreasonable effectiveness of mathematics" refers to:
(a) The complexity of mathematical equations.
(b) The surprising accuracy of mathematics in describing the physical world.
(c) The limitations of mathematical models.
(d) The difficulty of understanding mathematical concepts.
12. Wigner argues that there's no fundamental reason why:
(a) Mathematics should be so difficult to learn.
(b) Mathematical laws should change over time.
(c) The constants and laws of the universe should be possible to express in written formulas.
(d) Mathematics should be applicable to all fields of study.
13. The color red thought experiment illustrates the problem of:
(a) The subjective nature of sensory experiences.
(b) The objective reality of physical phenomena.
(c) The limitations of scientific instruments.
(d) The universality of human perception.
14. Richard Hamming suggests that some scientific discoveries originated from:
(a) Purely mathematical reasoning, later confirmed by observation.
(b) Accidental observations in the real world.
(c) Government funding for scientific research.
(d) Collaborative efforts between scientists.
15. Galileo's law of falling bodies was, according to the video, proven through:
(a) Real-world experiments with precise measurements.
(b) Imagined, controlled scenarios and mathematical reasoning.
(c) Ancient Greek philosophical principles.
(d) Observation of natural phenomena over long periods.
16. The video suggests that experiments proving mathematical laws are often imperfect because:
(a) They are subject to human error.
(b) Real-world conditions can never be perfectly controlled.
(c) Mathematical equations are inherently flawed.
(d) Scientific funding is often inadequate.
17. Einsteinian physics demonstrated that:
(a) Newtonian physics was completely wrong.
(b) Newtonian physics was incomplete.
(c) Quantum physics was unnecessary.
(d) Mathematics was irrelevant to physics.
18. Schrodinger's cat illustrates the idea that:
(a) Cats are unpredictable creatures.
(b) Quantum mechanics is easily understood.
(c) Reality at the quantum level can be paradoxical.
(d) Experiments always confirm mathematical predictions.
19. The video concludes that for most daily purposes:
(a) Abstract mathematical concepts are essential.
(b) The human brain understands reality without complex mathematics.
(c) Newtonian physics is irrelevant.
(d) Quantum physics governs our everyday experiences.
20. According to the video, even if maths isn't real:
(a) We should abandon scientific inquiry.
(b) Everything is going to be fine.
(c) We should focus on practical applications of science.
(d) The universe is fundamentally chaotic.
Follow-up 4: Learning the specific Language _ PDF Presentation on Campus.
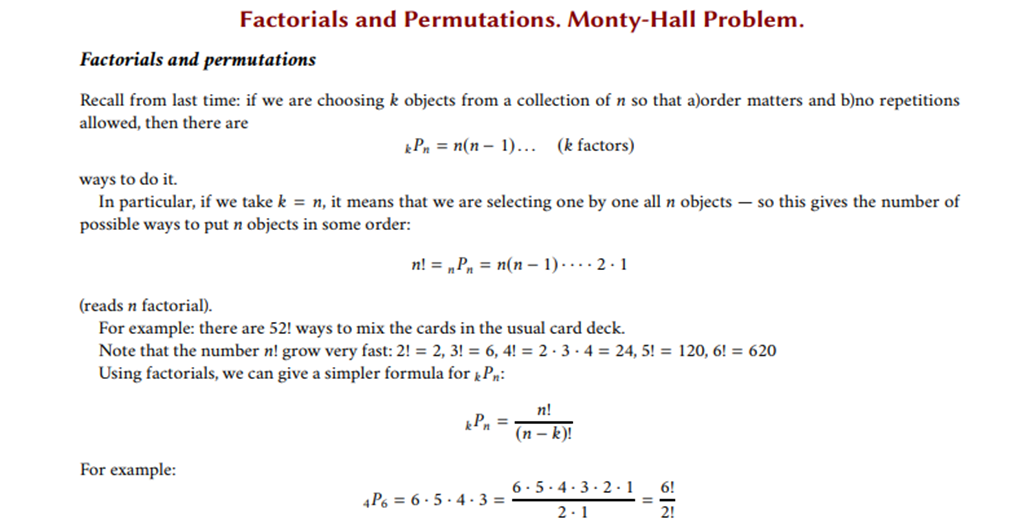
How to Read these Mathematical Operations in English:
Factorials and Permutations
"n!" is read as "n factorial."
"kPn" is read as "permutations of k out of n" or "n permute k."
Example: "6!" is read as "six factorial," which means "six times five times four times three times two times one."
The formula for permutations: is read as: "The number of permutations of k objects out of n is equal to n factorial divided by the factorial of n minus k."
Example: is read as: "Permutations of four out of six is equal to six factorial divided by two factorial."
Monty-Hall Problem
Suppose you’re on a game show, and you’re given the choice of three doors: Behind one door is a car; behind the others, goats. You pick a door, say No. 1, and the host, who knows what’s behind the doors, opens another door, say No. 3, which has a goat. He then says to you, “Do you want to pick door No. 2?” Is it to your advantage to switch your choice?
A car and two goats are arranged behind three doors, with the position of the car being random and uniformly distributed among the three doors, and then the player initially picks a door. Assuming the player’s initial pick is Door 1 (the same analysis applies for any other door the player picks), then according to the problem statement in which the host must always open a door after the player chooses one, then there are three equally likely cases:
• The car is behind Door 3 and the host must open Door 2: Probability = 1/3
• The player originally picked the door hiding the car. The game host must open one of the two remaining doors randomly, so there are two subcases
– The car is behind Door 1 and the host opens Door 2: Probability = 1/6
– The car is behind Door 1 and the host opens Door 3: Probability = 1/6
• The car is behind Door 2 and the host must open Door 3: Probability = 1/3
These cases and the total probability of each of them occurring are shown in the figure below. If the host has opened
Door 3 switching wins in the 1/3 case where the car is behind Door 2 and loses in one 1/6 subcase where the car is behind
Door 1, hence switching wins with probability 2/3.
Monty Hall Problem (how to read these numbers / operations in English):
"1/3" is read as "one-third."
"2/3" is read as "two-thirds."
Probability statements:
"The probability that the car is behind door 3 is one-third."
"Switching doors increases the probability of winning to two-thirds."
Classwork:
1. A group of 6 club members always dine at the same table in the club; there are exactly 6 chairs at the table. They decided that each day, they want to seat in a different order. Can they keep this for a year? Two years?
2. How many ways are there to seat 15 students in a classroom which has 15 chairs? If the room has 25 chairs?
3. A small theater has 50 seats. One day, all 50 seats were taken – but the people took seats at random, paying no attention to what was written on their ticket.
a. What is the probability that everyone was sitting in the right seat (i.e., the one written in his ticket)?
b. What is the probability that no person was sitting in the right seat?
4. A puzzle consists of 9 small square pieces which must be put together to form a 3 × 3 square so the pattern matches (this kind of puzzles is actually quite hard to solve!). It is known that there is only one correct solution. If you started trying all possible combinations at random, doing one new combination a second, how long will it take you to try them all?
5. a. How many 5s are there in the prime factorization of the number 100!? How many 2s?
b. In how many zeroes does the number 100! end?
6. 10 people must form a circle for some dance. In how many ways can they do this?
7. Here is another question similar to the Monty Hall question discussed today. You know that the family next door has two children. You met one of them, and he is a boy. What is the probability that the other one is a boy, too?